We don’t know how much ribbons cost but we can work out where the decimal point goes.
We add a zero to the end of the cost of ribbons, that gives us the cost of 10 inches. The decimal point moves one place to the right.
We need 1/100 of this, so the decimal point moves two places to the left because 100 has two zeroes.
One place to the right plus 2 places to the left gives us one place to the left. Therefore, since the cost of bows and ribbons is the same, the final result is that the decimal point is moved one place to the left of the cost of an inch of ribbon. For example, if the cost of ribbons is 12 cents per inch, which is the same as 12.0 cents, so the final answer is 1.20 cents. Your chart would be like a number line 1-2=-1, where positive is to the right and negative is to the left, so one place to the left.
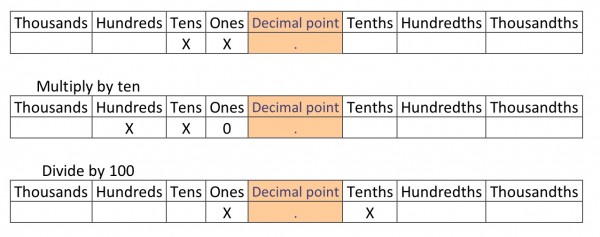
In this chart I have used XX as the cost of ribbons (and bows) per inch. You can use actual numbers in place of XX to see what happens. When XX moves to the left, the decimal point appears to move to the right, and when XX moves to the right, the decimal point appears to move to the left. Any gaps to the immediate left or right of the decimal point are filled with a zero to show that there is no value in that place. Replace XX with 12 (for example) to see what happens. Then replace XX with 08 (another example where cost is 8c or $0.08) and see what happens.