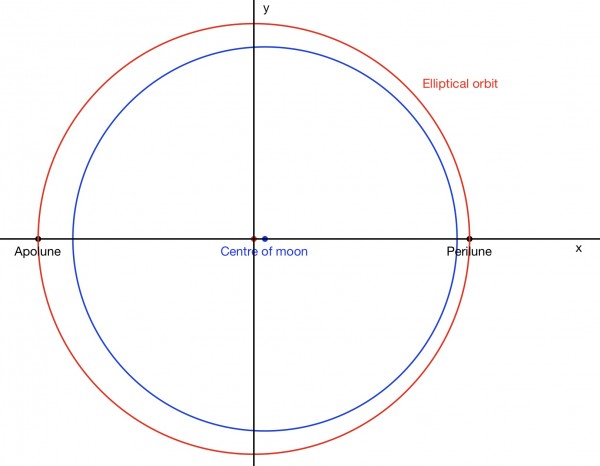
The radius of the moon is 1728km, so the perilune is 1728+110=1838km from one focus and the apolune is 1728+314=2042km from the same focus. The general equation of an ellipse is x²/a²+y²/b²=1.
Therefore, when y=0, x=±a. The length of the major axis (the distance between perilune and apolune) is 2a=1838+2042=3880km, making a=3880/2=1940km. f²=a²-b² where f is the focus, so b²=a²-f². But f is the distance of the focus from the centre of the ellipse. We know a, the semi-major axis so f=1940-1838=102km and b²=1940²-102²=3753196..
The equation is x²/3763600+y²/3753196=1. The centre of the ellipse is at (0,0), as shown by the red point.