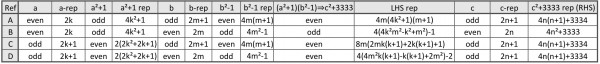
The following proof is based on equality of parity (odd/even). The table summarises the algebraic processes involved. The Ref column is just to identify the rows of the table as referred to below.
The idea is to show that there are no integer solutions related to the rows A-D, using basic algebra. In the table, k, m and n are positive integers (natural numbers). All even numbers can be represented by the expression 2x, where x is an arbitrary integer, and all odd numbers by 2x+1.
We look at the parity of the left-hand side (LHS) of the given expression. The parity of the right-hand side must match that of the LHS.
For each row A-D, the 10th column of the table gives the LHS parity and implies the RHS parity. These rows cover the 4 possible combination of the parities of a and b. Take row A, for example. In this row a is even and b is odd. In the other columns, the parities of a², a²+1, b², b²-1 and the whole LHS are derived from the parities of a and b. For row A, the LHS parity is even, and this implies the RHS parity, and this determines the parity of c.
By equating the parities of LHS and RHS we arrive at algebraic expressions. To prove that no integers a, b and c satisfy parity equality, we look at the expressions for each row and try to prove that that there are no solutions for a, b, c.
ROW A
The expression for LHS in column 11 is a multiple of 4; but if we divide the expression in column 14 by 4, we can see that 3334/4=1667/2, which is clearly not an integer. Therefore, row A has no integer solutions.
ROW B
The equation can be rewritten 4(4k²m²-k²+m²)=4n²+3334, by taking the -1 from LHS and adding it to 3333. Since 4 does not divide exactly into 3334, there can be no integer solutions.
ROW C
Here we introduce two new variables, k₁ and n₁:
k₁=k(k+1)/2, and, because within two consecutive integers, one is odd and the other is even, so their product is always even, making k₁ an integer. Similarly, integer n₁=n(n+1)/2. We can write 2k₁ and 2n₁ in place of their expressions:
8m(4mk₁+4k₁+1)=8n₁+3334.
LHS is a multiple of 8, but RHS is not, because 3334/8=1667/4, not an integer.
There can therefore be no integer solutions.
ROW D
Applying the same substitutions as for row C and adding 2 to both sides of the equation we get:
4(8m²k₁-2k₁+2m²)=8n₁+3336,
8(4m²k₁-k₁+m²)=8n₁+3336.
Divide through by 8:
4m²k₁-k₁+m²=n₁+417.
From this, n₁=4m²k₁-k₁+m²-417=k₁(4m²-1)+m²-417.
At first sight we might think that there are multiple solutions for k₁, m and n₁, from which a, b, c can be derived. However, k₁ and n₁ are not arbitrary, because they are derived from k(k+1)/2 and n(n+1)/2, where k and n are arbitrary integers. With k, n starting at 1 we can create a series:
1, 3, 6, 10, 15, 21, ...
More to follow...
Explanation and solution to follow in due course...