Let's see how many ways 4 digits can add up to 28. We start with the highest digit 9 and subtract it from 28: 28-9=19. Now we subtract the next highest digit 8: 19-8=11. The 2 remaining digits must add up to 11: 5+6, 4+7. So we have two numbers to start with: 5689 and 4789. But we can rearrange each of these in 24 different ways. That gives us 48.
In fact, the largest number we can make with the digits is 9876 whose digits sum to 30. So to sum to 28 we have only the digits 9, 8, 6 and 5 and 9, 8, 7 and 4. Nevertheless, we'll look at a general method for solving the problem.
So that means we only need to work out 4-digit numbers where the digits are in order and add up to 28. When we've found out how many there are we simply multiply by 24 to find out how many 4-digit numbers there are in total.
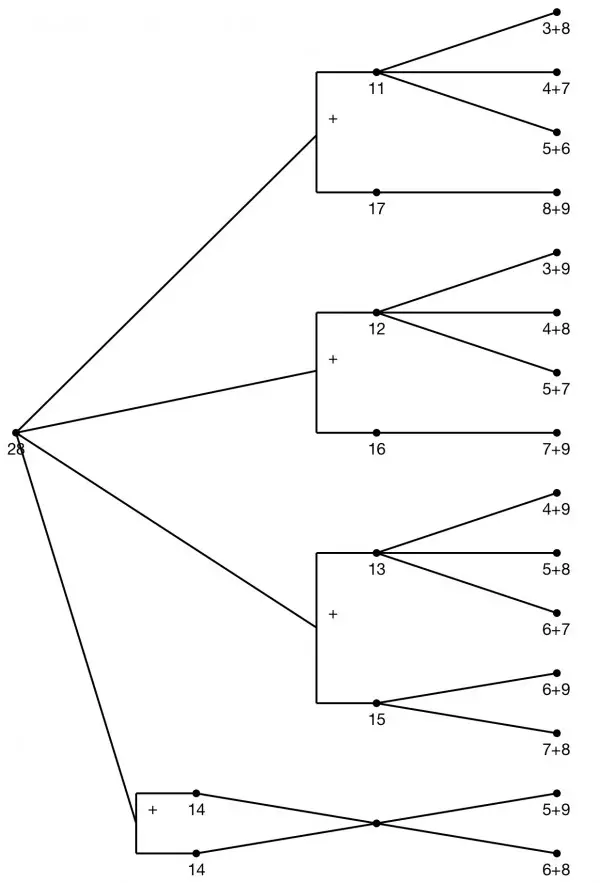
The tree diagram is supposed to help you work out the 4-digit numbers whose digits sum to 28. It shows how we can take the sum of two pairs of digits and add their sums. For example, 28=11+17, shown at the top of the diagram. Then it shows how 11 and 17 can be the sum of single digits.
On the extreme right (the leaves of the tree) you can see what digits make up the sums. We need the leaves that have no repeated digits. At the top of the diagram we have 3 alternative leaves for the sum of 11: 3+8, 4+7 and 5+6. And for 17 we have only 8+9. So we can't have 3+8 with 8+9 because 8 is repeated, but we can have both of the alternatives. That gives us 4789 and 5689. Using this method we can find any others that work. But we always come up with the same set of digits, and we identified them earlier. So there are 48 numbers (rearrangements of these numbers).